Are you looking for an answer to the topic “what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+9y^2=36“? We answer all your questions at the website barkmanoil.com in category: Newly updated financial and investment news for you. You will find the answer right below.
Keep Reading
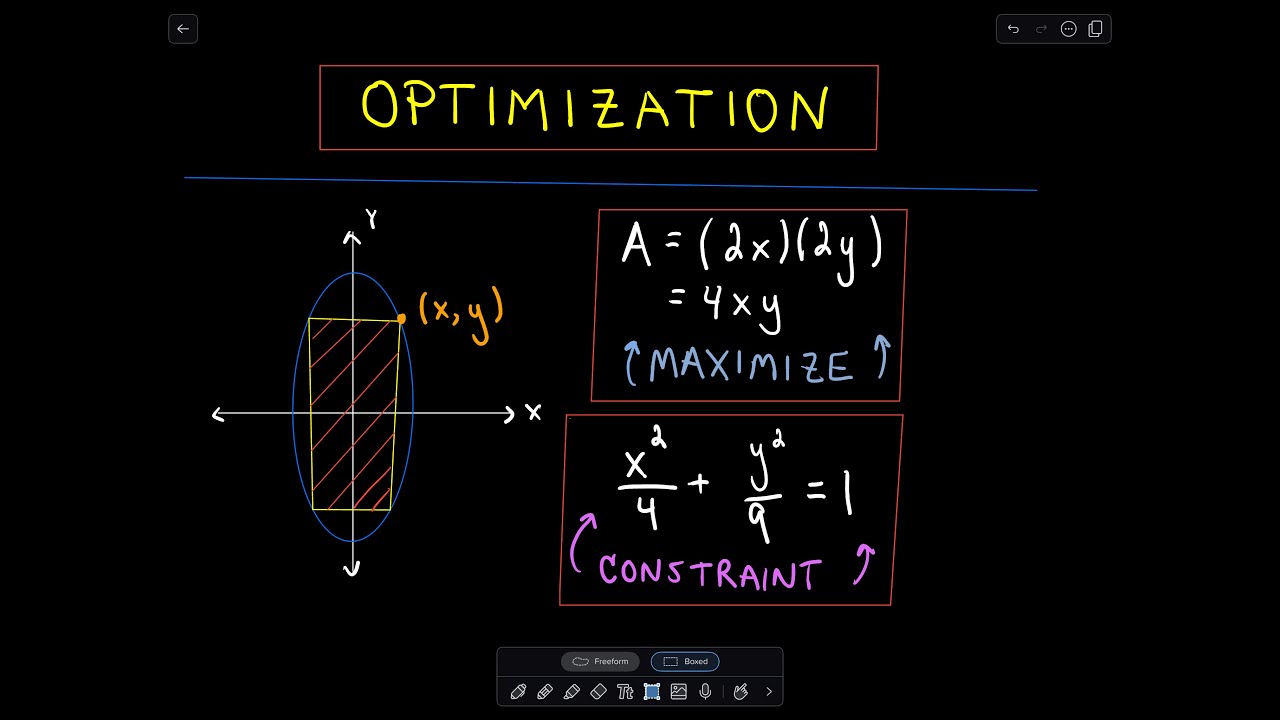
How do you find the largest area of a rectangle inscribed in an ellipse?
Let the upper right corner of the rectangle has co-ordinates (x, y), Then the area of rectangle, A = 4*x*y. Setting this to 0 and simplifying, we have y2 = b2x2/a2. Thus, y2=b2 – y2, 2y2=b2, and y2b2 = 1/2.
How do you find the area of a rectangle with an ellipse?
- Let, the length of the major axis of the ellipse = 2x and the length of the minor axis of the ellipse = 2y.
- From the diagram, it is very clear that, 2x = l. 2y = b.
- So, Area of the ellipse = (π * x * y) = (π * l * b) / 4.
Calculus Optimization #9: Largest Rectangle Inscribed in Ellipse
Images related to the topicCalculus Optimization #9: Largest Rectangle Inscribed in Ellipse

What is the area of the largest rectangle that can be inscribed in a circle of radius r?
Answer: The maximum area of a rectangle inscribed in a circle of radius ‘r’ is: 2r².
What is the area of the largest rectangle that can be inscribed in a semi circle of radius 10?
A rectangle is inscribed in a semicircle of radius 10 cm. What is the area of the largest rectangle we can inscribe? Amax = xw = (5 / 2)(10 / 2) = 100 Page 7 A poster is supposed to have margins of 1 inch on the left and right and 1.5 inches on top and on bottom. The printed area is supposed to be 54 square inches.
How do you find the area of the largest rectangle that can be inscribed in a semicircle?
Let r be the radius of the semicircle, x one half of the base of the rectangle, and y the height of the rectangle. We want to maximize the area, A = 2xy. Thus, the base of the rectangle has length = r/√2 and its height has length √2*r/2.
What will be the largest area of rectangle that can be inscribed in a circle of diameter D?
Now the area (A) of the rectangle is length multiplied by breadth. Hence x = y =r√2 thus it forms a square with maximum area. So the rectangle of maximum area inscribed in a circle is a square.
What is the maximum area of a rectangle inscribed in a right triangle?
Therefore it is the case that if a rectangle is inscribed inside a right-angled triangle in this way, its greatest area will be exactly half that of the triangle. One of the first things we must do when taking an algebraic approach is to decide which length in the diagram to consider as our variable.
See some more details on the topic what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+9y^2=36 here:
What is the area of the largest rectangle that can be inscribed …
A=12. Explanation: 9(x2)+4(y2)=36≡x24+y29=1. The problem can be posed as: Find Max xy or equivalently Max x2y2 such that. x24+y29=1.
What Is The Area Of The Largest Rectangle That Can … – Ask-rk
Thus the maximum area of a rectangle that can be inscribed in an ellipse is 2ab sq. units. Furthermore, what is the area of the greatest rectangle that can …
Find the area of largest rectangle that can be inscribed in an …
The vertices of any rectangle inscribed in an ellipse is given by (±acos(θ),±bsin(θ)). The area of the rectangle is given by A(θ)=4abcos(θ)sin(θ)=2absin(2θ).
Which of these rectangle has biggest area?
As shown with the algebraic proof using differentiation, the square of 25m x 25m gives the biggest area. If you wanted a non-square rectangle, then the closer the sides are to equal, the bigger it would be.
What is the equation for ellipse?
The equation of an ellipse written in the form (x−h)2a2+(y−k)2b2=1. The center is (h,k) and the larger of a and b is the major radius and the smaller is the minor radius.
Optimization Question – Rectangle Inscribed in an Ellipse
Images related to the topicOptimization Question – Rectangle Inscribed in an Ellipse

What is the largest rectangle that can fit in a circle?
The rectangle of largest area inscribed in a circle is a square.
When a rectangle is inscribed in a circle?
A circle inscribed in a rectangle touches the larger side of the rectangle with its ends i.e. the length is tangent to the circle. A rectangle inscribed in a semicircle touches its arc at two points. The breadth of the rectangle is equal to the diameter of the circle.
At which points on the curve Y 1 40x 3 − 3x 5 does the tangent line have the largest slope?
6. At which points on the curve y =1+40×3 – 3×5 does the tangent line have the largest slope? The points which maximizes the tangent line slope are therefore (2, 225) and (-2, -223).
What is the shape of the figure if rectangle is inscribed in a fixed circle?
Thus it is a square.
What is the area of the largest triangle that can be inscribed in a semicircle of radius r?
Therefore, the area of a largest triangle that can be inscribed in a semicircle of radius r is r² square units.
What is the area of the largest square that is inscribed in a semicircle of radius 10 cm?
Detailed Solution
∴ The area of the largest square is 392 cm2.
What is semicircle formula?
The area of a semicircle is half of the area of the circle. As the area of a circle is πr2. So, the area of a semicircle is 1/2(πr2 ), where r is the radius. The value of π is 3.14 or 22/7.
Example 1: Optimization: Finding the largest rectangle that can be inscribed in an ellipse.
Images related to the topicExample 1: Optimization: Finding the largest rectangle that can be inscribed in an ellipse.

Why is x2 y2 r2?
x2 + y2 = r2 , and this is the equation of a circle of radius r whose centre is the origin O(0, 0). The equation of a circle of radius r and centre the origin is x2 + y2 = r2 .
Is the area of a rectangle 2xy?
In this way the area of the rectangle is given by A = 2xy. 2 = r2 > 0. Hence, the maximum area of the rectangle is r2.
Related searches to what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+9y^2=36
- ellipse inscribed in a rectangle
- what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+y^2=16
- what is the area of the largest rectangle that can be inscribed in the ellipse 4x2y216
- find the dimensions of the largest rectangle that can be inscribed
- the area of the largest rectangle that can be drawn
- maximum perimeter rectangle in an ellipse
- find the dimension of the largest rectangle that can be inscribed in the ellipse
- maximum area of a rectangle inscribed in an ellipse calculator
- find the area of ellipse 4×2 9y2 36
Information related to the topic what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+9y^2=36
Here are the search results of the thread what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+9y^2=36 from Bing. You can read more if you want.
You have just come across an article on the topic what is the area of the largest rectangle that can be inscribed in the ellipse 4x^2+9y^2=36. If you found this article useful, please share it. Thank you very much.